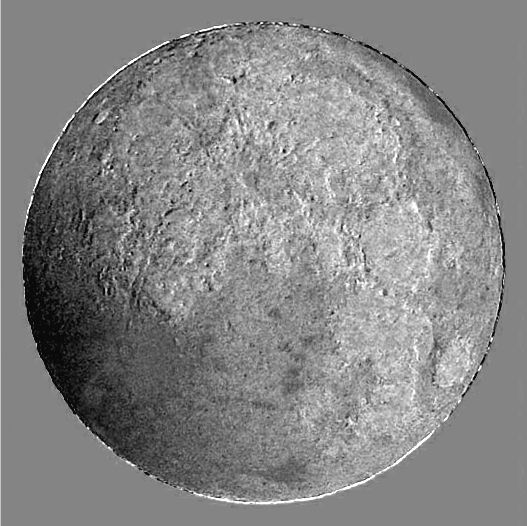
N
E
C
L
I
P
S
E
0
0
3
8
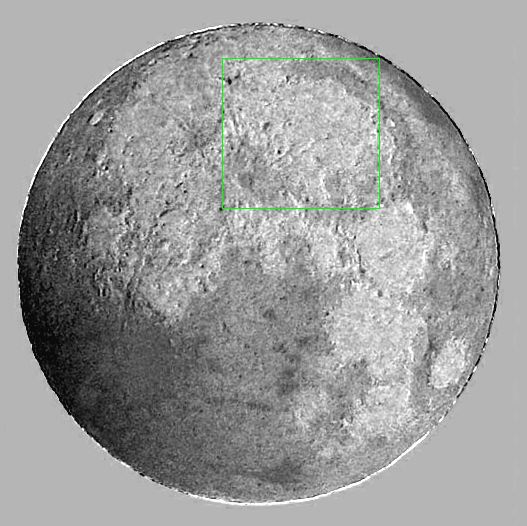
U
T



U
T
O
F
E
C
L
I
P
S
E
0
1
4
9
U
T



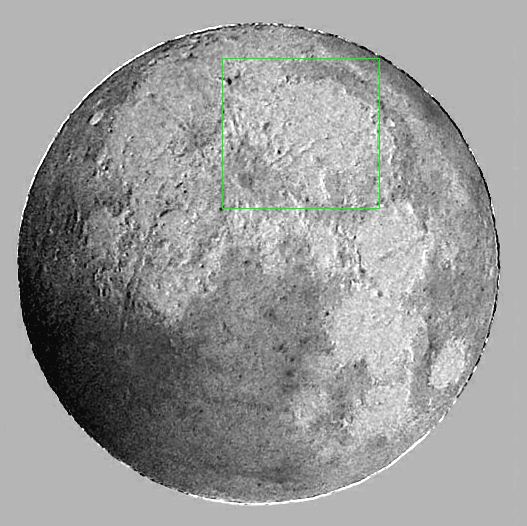
A
W
D
I
F
F
.
I
M
A
G
E
S


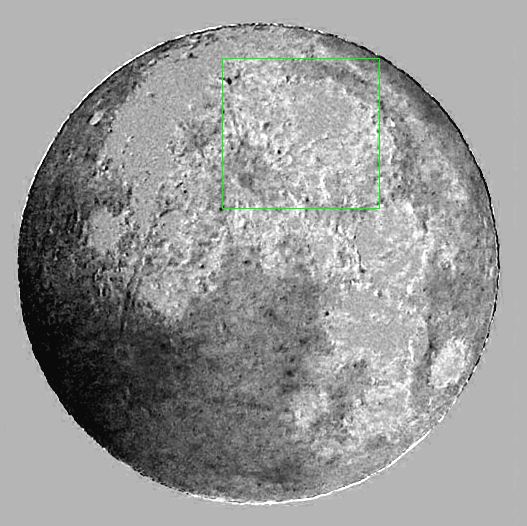
O
R
M
A
L
I
Z
E
D
D
I
F
F
.
I
M
A
G
E
S
| RED |
GREEN |
BLUE |
|
| I N E C L I P S E 0 0 3 8 U T |
 |
 |
 |
| O U T O F E C L I P S E 0 1 4 9 U T |
 |
 |
 |
| R A W D I F F . I M A G E S |
 |
 |
 |
| N O R M A L I Z E D D I F F . I M A G E S |
 |
 |
 |
