TIME-OF-DAY
THUNDERSTORM PROBABILITY
Since mid-totality in this region is at appx 1:50 UT (=local time as
Gabon is on GMT), the logical question to ask is: WHEN during the day
are these anticipated thunderstorms most prevalent (if they ar not
uniformly distributed)? These data themselves,
unfortunately, do not discriminate for time of day. Hence,
to answer this question, I then consulted an aviation weather archive
with a historical data record that is specifically germane in answering
this question. That archive has a web portal to access the
raw data (not yet reduced), as
reported in 1-1/2 hour increments w.r.t. thunderstorm activity, that
allows a detailed assessment of the situation.
In particular these data are reported from the weather station at the
Libreville airport. That is the WMI id station # 64500
(here:
http://weather.gladstonefamily.net/site/FOOL). The
web-interface for these data is here:
http://rp5.ru/Weather_archive_in_Libreville_(airport).
While contemporary data reported for the station at the Pt. Gentile
airport, historical data from that station is not available through
this archive. But, as spoken to earlier, as a statistical
estimator of expectation, the Libreville historical record should serve
as a very close proxy to the (unavailable) Pt. Gentil historical data.
You can go to the above link to access the Libreville historical
data. For convenience, I have a downloadable PDF file with all
entries over a one month period from mid-October to mid-November 2012
you can download or display ==>
HERE.
Other year data are available through the above web portal. Below
I have done just an analysis of the 1-month period centered the date of
the eclipse (Nov 3) for the most recent year (2012). I have
"looked through" prior years and -- without a detailed analysis -- they
all appear to tell a very similar story.
Most important is to note the data records in the columns labeled WW,
W1, and W2.
WW reports the "weather present" at the time of the corresponding data
record. Importantly this notes thunderstorms present (at the
airport) both with and without precipitation
W1 and W2, together report the same information but for 1-1/2 hours
prior to the current record.
So, together the time granularity of reporting the occurrence of
thunderstorms at the airport is every 1-1/2 hours
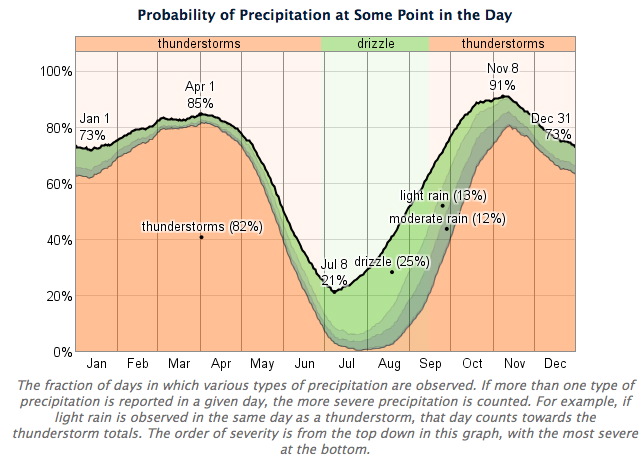
In terms of UTC these reports (for the times of interest) are:
10:00, 11:30, 13:00, 14:30, with totality at 13:50 UTC
Note: The data record is not fully complete, some entries for specific
times/dates are not recorded. For the 31 day period considered
Oct 18, 2012 - Nov 17, 2012 the actual number of dates for which data
were recorded are noted, and statistics computed only for enttries that
are made (not missing), in the table below.
Here is what these historical data w.r.t. thunderstorms at the airport
inform...
Oct 18, 2012 – Nov 17, 2012
Libreville Airport Station Thunderstorms
|
|
10:00 UTC
|
11:30 UTC
|
13:00 UTC
|
14:30 UTC
|
Days Reported
|
23 days
|
26 days
|
22 days
|
19 days
|
Days with T-STORMS
|
13 days
|
21 days
|
10 days
|
15 days
|
% DAYS WITH
T-STORMS
|
57%
|
80%
|
45%
|
80%
|
RELEVANCE TO
THE PROPOSED GABON ECLIPSE FLIGHT
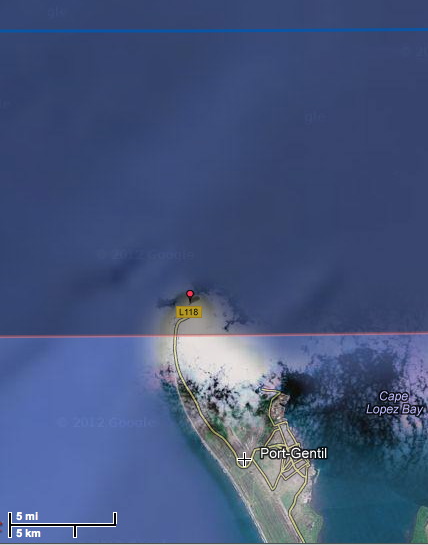
John has proposed a Port Gentil launched eclipse flight as depicted on
the graphic he provided below:
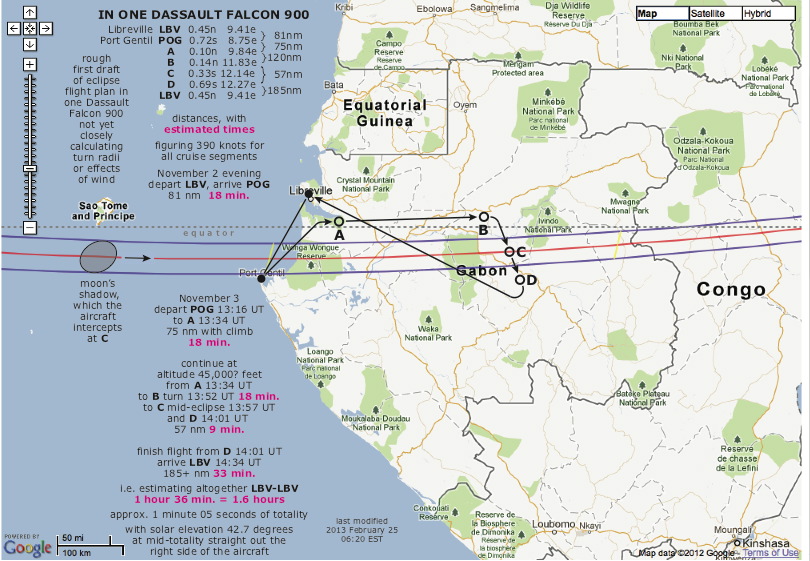
The above requires a "wheels-up" time no later than appx 13:16
UT. This is most closely matched in time for the historical data
records at 13:00 UTC. That indicates that, on average,
45% of the
time there are active thunderstorms at the airport, at, or close to
latest UTC of take-off and higher earlier.
Note this is an aviation weather, not flight operations, data
archive. The percentages derived above may not necessarily fully
correlate with flight delays (which could not be tolerated for an
eclipse flight) or cancellations due to in situ thunderstorms. I
have been unable to find such a historical fight operations database
for Gabon, and so now depart from "just the facts" and offer some
subjective commentary:
SUBJECTIVE COMMENTARY/ANALYSIS FOLLOWS:
Subjectively (without the benefit of flight operations historical
data), I posit it is conservatively appropriate to suggest that an
aircraft (in particular a small one such as a Falcon 900) would not
take off knowingly into an active thunderstorm. This may not be
"disallowed", but it certainly in not a great idea. In this I am
no expert, and invite any
professional jet pilots who may happen to read this (ROWLAND?) to
comment. I will just point you to F
AA
Advisory Circular 00-24B on Thunderstorms, in particular "%.
Hazards. A thunderstorm packs just about every weather hazard known to
aviation into one vicious bundle", 7.1 "Don't land or takeoff in
the face of an approaching thunderstorm. A gust front of low
level turbulence could cause loss of control", and other good
advise. And, more recent
FAA-P-8749-12
• AFS-8: in particular pretty succintly: "General Rules: Pilots
should observe the following rules for any flight eve potentially near
actual or possible thunderstorm activity: - Avoid all Thunderstorms.
...", and
this one that I
think that was written with eclipse chasers in mind: "Never let
compuslion tale the place of good judgement". My anecdotal
experience as a passenger is that quite
frequently when thunderstorms occur over the DFW airport (which are
frequent in summer), I end up often waiting hours on the tarmac or gate
(or sometimes overnight in a hotel) until they abate. I would
expect no different in Gabon as this is a safety issue.
John has suggested that (this est. 45% risk based on historical data)
could be mitigated in the case where a T-storm over the airport that
might cause a flight-delay is predicted ahead of time by an early
take-off - to get around/over it before it might cause a
flight-stoppage at the airport. In principle this sounds quite
reasonable, but the same data for times earlier than appx 13:00 UTC do
not support its likelihood of implementation. E.g., at
11:30 UTC, should an advisory of a likely ground-stop for 13:00 be
expected, there is an even higher (80%) chance of thunderstorms at that
earlier time as well. On any given day this scenario might
play out quite differentl, of course. Indeep it could be a highly
unusually gloriously clear day (but I would not count on that!).
This is simply what the historical record for this past year informs.
QUERY: A separate question, to John, unrelated to
expectation/implementation - but relative to COST is the
following. The charter quote John has obtained is for an appx 1.6
hour flight. If a ground-stop due to thunderstorms is predicted
for the ~ 13:00 (or encroaching earlier) take-off time, but possible to
obviate say with a take-off at 11:30 (if that happens to be one of the
20% times when there are not T-storms then) -- how much "extra" would
this cost? I.e., to buy-down that (small) amount of risk
mitigation with an "extra" appx hour and a half for the end-to-end
flight?
Detail: One could shorten the end-to-end time in the air by planning an
intercept due north (closest distance) to Port Gentil. With that
the minimum time in the air would be for the airport departure
maneuvers and climb to altitude (est 20 minutes), line-up and totality
run (est. 10 minutes), an descent landing (est 20 minutes), so 50
minutes end-to-end. HOWEVER, the time-criticality and concept for
front-end "margin" against T-storm risk remains the same. E.g.,
for this "minimum distance" flight a T.O. time of appx 13:10 UT would
still be needed, which does not change the buy-down of "up front"
margin for risk reduction, though the "back end" of the flight would be
shorter (if we could land as planned as well).
END:
SUBJECTIVE COMMENTARY/ANALYSIS
CUMULONIMBUS
CLOUD-TOPS AT EQUATORIAL LATITUDES
It is sometimes mistakenly thought that eclipse-flights with jets
fully (or nearly so) mitigate the risk of cloud. This *IS* the
case at polar and near polar latitudes where the topopause in the
summer (when solar eclipses are visible at high latitudes) drops to as
low as 9 km and is very rarely (if ever) higher than 12 km. THIS
is exactly what made TSE 2003, T008, and soon TSE 2015 flights so
appealing. Immediately below is what I wrote about years ago for
TSE 2008 (but generically applicable) and remains unchanged:
|
THE
"WEATHER" (acuna matata)
At high polar latitudes, such
as at
our 82.6° N point of mid-eclipse intercept, the tropopausal
boundary
between the troposphere below (where "weather occurs") and the
stratosphere has typical heights of only 6—9 km (compared to 12—17 km
at mid and low latitudes). Polar stratospheric (nacrecous) clouds are
extremely rare and only form at very low temperatures (< -78° C)
during the polar winter, making the probability of cloud-free eclipse
viewing nearly 100% at our flight altitude of 37,000 ft (~11.3 km) and
"baseline" observing location. Of course, we have the luxury (and
flexibility) for in situ retargeting of our viewing location if that is
required for any reason, however unlikely.
At this altitude and latitude,
aerosol scattering of sunlight by airborne particulates is extremely
low, giving rise to an exceptionally dark sky during totality, enabling
eclipse viewing with significantly enhanced image contrasts. Moreover,
the airmass along the line-of-sight to the Sun is significantly reduced
(by ~ 75%), resulting in exceptional sky transparency, greatly reduced
atmospheric turbidity, and better astronomical "seeing". |
This is not the case for equatorial eclipses where the topopause can
reach up, actually in some cases, to 18 km (=59,000 ft) -- and the tops
of equatorial cumulonimbus clouds can extend to the top of the
correspondingly high-altitude troposphere. John had prior
indicated that the service ceiling we can expect from the Afrijet
Falcon 900 is 47,000 ft (~ 14 km; which would make it a shoe-in for a
polar eclipse), but not necessarily so for an equatorial eclipse.
It may be possible to eek out another half km or so, but that is
getting into th "noise" for this discussion.
It should be noted that the same aviation weather historical archive
from which the probability of thunderstorms as a function of time of
day at the airport was derived also reports on the occurrence of
occurrence of Cumulonimbous clouds (with and
without high altitude anvils) in the same record (column "Cl").
The correlation with thunderstorms is extremely high - but those data
do not indicate cloud-type height. So, to assess that I consulted
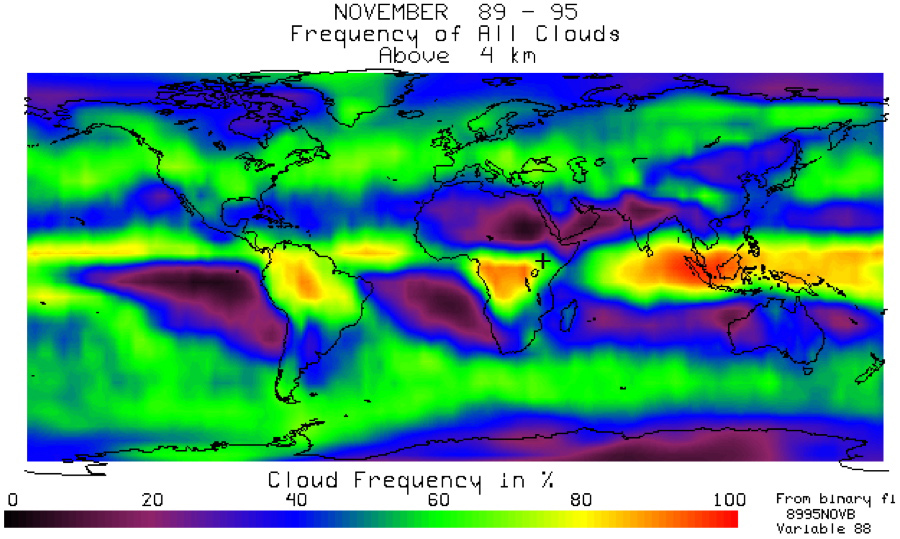
a global cloud-height map database maintained by the Space Science and
Engineering Center (SESC) of the University of Wisconson-Madison:
http://www.ssec.wisc.edu. I
reproduce the most relevant map below:
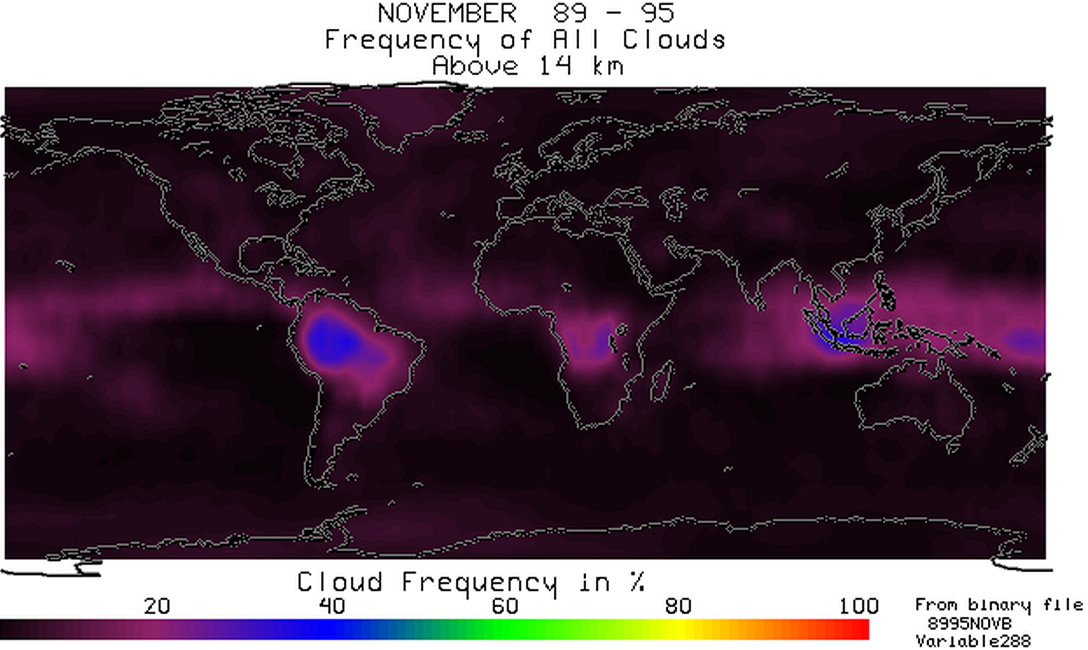
EXPECTATION FOR
CLOUD ABOVE AIRCRAFT OVER GABON
These above cloud frequency with height data do not inform on a
micro-scale the specific information over the Port Gentil airport, but
are of sufficient spatial resolution to inform of local probabilities
of very high altitude cloud at and above an aircraft flying in the
general region. I must say there there remains some uncertainty
as to the absolute calibration accuracy of these data, so there is some
uncertainty here - but the trends is clear. These data for
November inform that in the equatorial zone (where the tropopause is
highest) clouds persist at altitudes >= 14 km up up to ~ 20% of the
time over the Oceans, and up to ~ 40% of the time over central land
masses -- when clouds exist. Note this is the frequency of
occurrence at >= 14km
when clouds
exist - so in absolute terms one must assess when clouds
exist. Over Gabon, in November, clouds will (unfortunately) occur
~ 90% of the time. E.g., see below:
This is confirmed locally at the Libreville airport from the prior
noted aviation weather record for the time of day of interest for the
eclipse in the total cloud cover (N) records. The SESC map
informs that over inland coastal equatorial Africa (Gabon) cloud above
14 km can occurs ~ 20% of the time when clouds are present.
(Digression: It is interesting to note while this increases in central
equatorial Africa, this does not persist into Northern Kenya at
Lake Turkana). And the total sky cover map (and met data records)
indicate ~ 90% cloud cover. Taken together one then infers an
expectation that ~ 18% of the time when
flying over Gabon in November one may still have cloud higher than 14 km.
COMMENT: Given this quantitative risk assessment, it might make
more sense to consider a Gabon launched eclipse flight to go over the
Ocean (where this declines, see the SESC map) rather than inland.
However, as John separately points out, that cannot go too far from the
coast as he altitude of the Sun will become problematic for windows in
the falcon 900 aircraft.
GABON
FLIGHT WEATHER RISK SUMMARY
From the above, I objectively conclude that,
under the assumption that the immediate
presence of active thunderstorms at the airport would an aircraft
take-off, that there is a 45% or greater risk of the
Gabon-launched eclipse flight not getting off the ground in a requisite
time window to see the eclipse. This risk MIGHT be reduced
somewhat overall by allowing for a (significantly) earlier take-off
time to extend that time window for T-storm avoidance. E.g., as
early a talke-off as 11:30 would permit an aircraft to take-off under
the same assumption (and additional) 20% of the time at that time of
day. I do not do a cost trade as I do not have that
information.
Additionally, if the aircraft can tale-off between times of
thunderstorm activity at the airport (anticipated 55% of the time),
there then is an ~ 18% risk that the aircraft cannot get high enough to
overfly high-altitude CN cloud tops. This risk may be overstated
due to uncertain calibration of the SESC cloud frequency with altitude
maps. This ~ 18% risk could be reduced by planning a flight over
the Ocean rather than inland.
SNP (LAKE
TURKANA) GROUND-WEATHER RISK ABBREVIATED SUMMARY
As previously written to in emails, based on a combination of
in-situ historical weather reporting at Lodwar (as a proxy to nearby
SNP) and remote sensing satellite data, the derived
expectation value
for seeing totality from SNP unobscured by intervening cloud is 65%
+/-15% (i.e. a 35% risk of a "cloud out) from the
ground.
Here (immediately below to capture this information) is what I had
previously summarized,. This is all "detail" that can be skipped
if you read previously.
It is obvious to us that {on the
ground} the immediate Lake Turkana
area is the stand-out best location for climatological expectation of
least cloud cover along the line-of-site to the Sun (despite its only
12 degree at totality altitude) -- excepting the extreme eastern end of
the path with the Sun VERY low, in the Ogaden region of Ethiopia and at
sunset in western Somalia that we reject for these and other
reasons.
The usual "metrics" that we use to try to assess the probabilities for
unobscured eclipse viewing, in this case, lead to different (though
still "best") expectations. Some of those differences ar
understood,
but that understanding does not help close the uncertainties.
Let me summarize what we are currently working with, but also to point
you to a weather summary meteorologist and eclipse-chaser Jay Anderson:
http://home.cc.umanitoba.ca/~jander/tot2013/tse13intro.htm
In particular, of course, is the information on the Lake Turkana and
SNP region which is our focus. Let me call your attention first
to
"Table 1" entries for Lodwar that we use as a proxy for SNP as the
weather in general we expect to be quite similar. These tabulated
data
are derived from in situ, on the ground, measures from Lodwar, so are
in many respects "better" that what can be derived from remote sensing
with satellites (those are the maps, in particular Fig 2, which
is
discordant in part for that reason).
It is suggested that the column labeled "Percent of Possible Sunshine"
is the best (single) indicator, with the highest correlation, to
directly extract a probability of seeing the eclipse unobscured by
cloud. What this metric actually is, by definition is: "The
total
time that sunshine reaches the surface of the earth, expressed as the
percentage, of the maximum amount possible from sunrise to sunset with
clear sky conditions." In table 1 this is based on
early
November data averaged over many years, where the number of maximum
possible daylight hours is very close 12 hours. The data
analyzed
here suggest 80% (9.6 hours on average out of 12) with sunshine
reaching the surface of the Earth. Other historical data archive
sites
consistently report 9 hours if 12 (to the nearest hour) of 75%. I
am
not concerned over that 5% difference, but is it actually that
high?
This seems somewhat counter (higher) than a different method of
analysis that I will explain.
Additionally these (and other data discussed below) are not
discriminated by the time of day. What REALLY is of interest to
us is
the simple question: ow likely is it that we will se the eclipse (from
the ground) unobscured by intervening opaque clouds - with the eclipse
occurring an hour before sunset with the Sun 12 degrees above the
western horizon looking out over Lake Turkana? And, what,
actually,
are the prevalent cloud conditions then?
There is a different way to look at this, also reflected by this table
as follows. Reported here are the (average for early November
over
many years) breakdown - by relative percentage - of different types of
sky (cloud) conditions reported by in situ weather observers in
Lodwar. This does not, unfortunately, give actual cloud type or
height, but rather the sky condition w.r.t. obscuration as: Clear, Few,
Scattered, Broken, Overcast, and Obscured. The distinction from
individual observations (before averaging) is in how many eighths (or
"octas") of the sky is covered by cloud of any type. These data
can be
used to compute a "Calculated Cloudiness", separately from the
"Percentage of Possible Sunshine" which is a weighted average of the
different percentages of the sky conditions. In some detail (more
than
probably needed here) the categories (not my doing, defined decades ago
by NOAA and adopted globally) are as follows:
Details: "Clear is no cloud. (It is suspicious to me that for Lodwar
"Clear" is so low as only 1% in Table 1). "Few" is defined as “from
zero to 2 eights – any amount up to 2/8ths except for zero itself.
(This MAY be why "clear" is only 1%, since "few" also allow for 0
eigthts!). A perfect observer will observe 2 ½ eights as
either 2 or 3 (doesn't
matter which, as it’s the limit). Therefore, the range for the "Few"
category is from zero to 2.5 eights cloud cover. The mid range is
(2.5/8)*0.5 or 0.1563 (I transposed the 2 and 6 in the example I gave
you). Similarly, scattered lies from 2.5 eights to 4.5 eights
(formally, 3 and 4 eights). Broken lies from 4.5 eights to 8 eights.
8/8ths is overcast. Some of the categories are ranges, and some are
fixed values." -- source email
from Jay Anderson
As a note "In reality, an observer is never that exact, and most will
allow a small amount of cloud and still record clear, or have a few
tiny openings and call it overcast."
With the above quantification, one then computes the "Calculated
Cloudiness" which is in other places sometimes called the "cloud
amount" as a weighted average that is...
cloud amount= 0.1526*few + 0.4375*scattered +0.7813*broken
+overcast +obscured +partly obscured
Many eclipse chasers rely on this number, BUT, it is actually not
observationally well determined. It DOES provide a useful metric
to
intercompare different sites all measured in the same way, but not so
good for an "absolute calibration". Jay Anderson (our
meterologist
friend who prepared the linked table) has said:
{The Calculated Cloudiness} "is
merely a number for comparative
purposes. I could use the mid range of the formal definition, but the
difference would be very small. The main error lies in the fact that
cloud distributions are usually U-shaped {not at all randomly
distributed}, and so linear
estimates will have built-in biases".
So, with the above caveat to be heeded, the "calculated cloudiness" for
Lodwar is formally 51%, but may under- or over- estimate the actual
expectation. The separately determined "Percent Possible
Sunshine" of
75%-80% clearly paints a more optomistic picture. Neither may be
fully
accurate as an expectation value - and without knowing the biases a
rational thing to do is simply to average these two at about 65% with
then an uncertainty of about +/-15%.
But again, despite this uncertainty -- which says we may have about a
2:3 chance of seeing totality on the ground, this does not inform at
all if this the case an hour before sunset in particular.
ONE MORE THING (mostly for my colleagues). If you look,
particularly
at Fig 2 on Jay's web page, the hi resolution "cloud cover" (yet still
a DIFFERENTLY DERIVED metric) map this seems further discordant than
with the in situ observations - far worse. Indeed this, derived from
satellite remote sensing data over 24 years - and specifically using
only AFTERNOON (more germane) data suggests for Lake Turkana about 65%
cloud cover. THIS, however, is NOT the same as the expectation
for
seeing totality - as this is for ANY type of cloud, distributed in the
sky in ANY manner Jay expressed this web page as (I add emphasis
in
bold):
"The satellite-derived cloud cover
charts are not exactly comparable to
measurements taken from the ground {Table 1} by humans and machines.
Observers and machines record from one spot, with an oblique view
toward the surrounding horizons. Satellites observe from altitude, but
also have oblique views toward the horizon, which lies much farther
away than for a ground observer. Machines see only the cloud directly
overhead and assemble areal measurements by keeping track of how the
overhead sky changes with time. Detection of cloud from satellites
relies on complex algorithms that compare infrared- and visible-light
radiation measurements to make a judgment about the extent of
cloudiness in the image pixels. Both ground-based and space-based
observations are concerned with cloud amount, while the important
parameter for eclipse-watchers is the opacity of the cloud - does it
block the Sun? In the extreme, a sky that is covered by transparent
cirrus cloud could be observed as overcast (in actual fact, it would be
recorded as "thin overcast") though it provides little impediment to
viewing an eclipse. The upshot is that satellite and surface-based
observations are probably biased to report a heavier cloud cover than
actually exists. The cloud charts in Figure 1 and 2 should then be used
to compare sites with each other rather than to derive an exact
probability of seeing the eclipse. That statistic is best represented
by the percent of possible sunshine in Table 1." |
SNP (LAKE
TURKANA) REDUCING WEATHER RISK WITH UNPRESSURIZED AIRCRAFT
This est. ~ 35% +/- 15% risk of cloud out from SNP, Lake Turkana is
reduced with a much less optimal ("last ditch") observation by air from
the Caravan 208B aircraft at ~ 12.5 kft (~ 4km). This aircraft
will not
be able to get over cloud above that level. The expectation value
for that somewhat uncertain but is likely to be ~ 60% for ALL
types of cloud types (see below) including both optically
thin/scattered, and opticaly thick/broken cloud.
The expectation for complete overcast skies is only 0.6% from the
Lodwar records, but may be under-reported. However, from the
historical precipiation record we might expect 2 days per month (6%)
with thunderstorms (presumably inclusive of the separately reported
overcast conditions) that would disallow the use of the aircraft backup
scenario. I.e, there is about a 6% probability that if we geel
the need to attempt observation by air (by expectation 35% probability)
we would not be able to do so.
Otherwise, during the 29% of the time when there is cloud for
which we might elect to take to the air and are able, we probably will
have broken to
scattered conditions. To assess the liklihood of such cloud in
the line-of-site to the Sun at heights above the aircraft we use as a
proxy for the average opacity of the different mix of cloud typesas
linear combination weigted mean of total cloud amount of 51%.
This leads to an expectation of frequency*cloud_amount = 30%.
I.e. 70% of the time that we would take to the air (29% expectation) we
likely could find a clear site-line to the Sun.
Summary:
Expectation of seeing the eclipse from the ground: 65% +/- 15%
Expectation of cloud-out with inability to fly: 6%
Expectation of "need" to fly: 29%
Expectation of seeing totality fron the air 12.5kft (in clear
skies or optically thin clouds) = 70%